How To Construct A Polynomial Function
Key Terms
o Polynomial function
o Subscript
o Coefficient
o Degree (of a polynomial)
o Nonlinear function
o Quadratic function
o Parabola
o Factor
o Quadratic formula
Objectives
o Recognize a polynomial function
o Know how many solutions a polynomial equation has
o Learn how to gene quadratic expressions
o Know how to utilise the quadratic formula
Polynomial Functions
Some other type of office (which actually includes linear functions, equally we volition run across) is the polynomial. A polynomial role is a role that is a sum of terms that each have the general form axn , where a and due north are constants and x is a variable. Thus, a polynomial function p(ten) has the following full general course:
![]()
Notation that we employ subscripts with the a factors (also referred to as coefficients) to brand the representation of the role more than compatible and lucid. When the function has a finite number of terms, the term with the largest value of n determines the degree of the polynomial: we say that the part is a polynomial of caste due north (or an n thursday degree polynomial). Thus, a polynomial of caste north can exist written as follows:
![]()
Observe, so, that a linear function is a first-caste polynomial:
![]() → f(x) = mx + b
→ f(x) = mx + b
Polynomials of a caste college than one are nonlinear functions; that is, they do not plot graphically equally a directly line. Instead, polynomials can have any item shape depending on the number of terms and the coefficients of those terms. Finding the zeros of a polynomial function (remember that a zero of a role f(x) is the solution to the equation f(x) = 0) can be significantly more complex than finding the zeros of a linear office. For simplicity, we will focus primarily on second-caste polynomials, which are also called quadratic functions.
Quadratic Functions
A quadratic function is a polynomial of degree ii. Because information technology is common, we'll use the following notation when discussing quadratics:
f(10) = ax ii + bx + c
Let's take a await at the shape of a quadratic function on a graph. We'll merely graph f(x) = x 2.
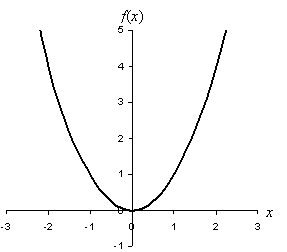

The graph shows that the office is obviously nonlinear; the shape of a quadratic is really a parabola. The quadratic function can be oriented either up (when a > 0, as in the above graph) or down (when a < 0), and it can be translated to any position in the plane (through variation of b and c). Note also that, depending on its location, the parabola tin cross the ten-centrality in two places, in one identify (as in the to a higher place graph), or nowhere at all. Mostly, however, a quadratic equation has two solutions, which may or may non correspond to the same number. Furthermore, the solutions to a quadratic equation may be circuitous numbers. (In fact, it is generally the case that an equation consisting of a polynomial of degree n has n solutions.) In that location are 2 essential approaches to solving a quadratic equation: factoring and the quadratic formula.
Solving Quadratic Equations: Factoring
A solution to whatsoever equation f(x) = 0 is the value or values of x for which f(x) is zero (that is, for which information technology crosses the x-centrality). Permit's look at the case quadratic function in a higher place:
f(x) = 10 2 = (ten)(10)
What we take done hither is factor the original expression. We can now see that this quadratic function has two zeros, both of which are at x = 0. Just what if the function is more complicated? Let'south offset consider the following general quadratic expression. We can aggrandize the expression by carefully applying the rule of distributivity.
f(x) = (px + q)(rx + s) = px(rx + southward) + q(rx + due south) = prx 2 + pxs + qrx + qs
f(x) = prx 2 + (ps + qr)x + qs
Annotation that this is fundamentally the same form as f(x) = ax 2 + bx + c, but different names are used for the coefficients. The zeros of the part are the 10 values for which either factor is equal to zero-thus, we tin can run across that there are generally ii solutions to a quadratic. We tin find these solutions by setting each factor equal to zero and solving for x. Of course, going from the factored course to the standard form is much more difficult than the reverse process, merely in many cases the factored grade can be institute without too much difficulty. The process of factoring a quadratic role is normally a procedure of trial and fault, simply with practice, yous tin learn to spot how to factor some quadratics.
Practice Trouble: Factor the expression 10 2 - 4.
Solution: This is our first problem involving factoring; start with what yous know. Nosotros can write a quadratic expression as follows:
(px + q)(rx + s) = prx 2 + (ps + qr)10 + qs
More simply, we can assume p = r = i and write
(x + q)(x + southward) = x 2 + (s + q)x + qs
At present, we see that s must be equal to –q and that the production of south and q must be –four.
due south + q = 0
southward = –q
And,
sq = –4
–s 2 = –four
south 2 = 4
Now, southward can exist either 2 or –2, simply information technology can merely be one or the other. Allow'due south merely selection i: southward = ii. And so, q = –2. Let's write the new expression and cheque our result:
(x + 2)(x – two) = x(ten – 2) + 2(10 – ii) = x 2 – 2x + 2x – 4 = 10 two – 4
This result checks out.
Practice Trouble: Factor the expression x ii + 3x + two.
Solution: We tin follow the same approach here as in the previous problem: our solution should have the following form:
(x + a)(x + b) = 10 2 + (a + b)x + ab = 10 two + 3x + 2
So, we know that ab = 2 and a + b = 3. Here, trial and error is the best road. By inspection, a = 1 and b = 2 satisfies these equations.
ab = (i)(2) = two
a + b = 1 + 2 = 3
b = two
a = i
The factored form is thus (ten + 1)(x + 2). (Annotation that the zeros of the function are and then x = –1 and x = –2.)
Practice Problem: Gene the expression x ii + 1.
Solution: Here we run into a slight problem. Permit's take a look.
(10 + q)(x + due south) = x two + (s + q)x + qs = x 2 + 1
We see that q = –southward, just too qs = 1.
qs = –s 2 = 1
s 2 = –1
southward = ± ![]()
Thus, we see that complex numbers can be involved in factors and zeros of quadratic functions. We can nevertheless handle this problem recalling what nosotros already know about circuitous numbers.
s = i
q = –i
So, the factored expression is (ten + i)(10 – i). Allow's expand this just to check.
(ten + i)(ten – i) = x 2 + ix – nine – i 2 = ten 2 – (–ane) = x 2 + 1
The zeros of this expression are and then i and –i. (As it turns out, circuitous solutions be when the graph of the quadratic function does not cross the 10-axis anywhere--effort graphing the expression in this problem to see.)
Solving Quadratic Functions: The Quadratic Formula
A more than general and straight way to observe the zeros of a quadratic function (and, also, to find the factors) is through the quadratic formula. Nosotros volition not derive the quadratic formula hither, but suffice it to say you can derive it using algebra. Given a quadratic part ax ii + bx + c, the zeros of the function are at
ten = ![]()
Exercise Problem: Find the solutions to the equation ten 2 – 4 = 0.
Solution: We can use the factoring approach, as nosotros did in a previous practice trouble, or we can use the quadratic formula with a = 1, b = 0, and c = –4. Let's endeavor this latter arroyo to compare.
x = 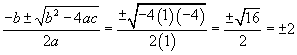
The solutions to the equation are so x = 2 and x = –ii. In some cases, the utilise of the quadratic equation is faster, even though factoring of the quadratic expression is still an option.
Source: https://www.universalclass.com/articles/math/algebra/how-to-solve-polynomial-functions.htm

0 Response to "How To Construct A Polynomial Function"
Post a Comment